Which Of The Following Is Not One Of The Function S Of Money: Coursehero

Sometimes, we come across a office that requires more than i formula in order to obtain the given output. For instance, in the toolkit functions, we introduced the accented value function

$f\left(ten\correct)=|x|$

. With a domain of all real numbers and a range of values greater than or equal to 0, absolute value can be defined as the magnitude, or modulus, of a real number value regardless of sign. It is the distance from 0 on the number line. All of these definitions require the output to exist greater than or equal to 0.

If we input 0, or a positive value, the output is the same equally the input.

$f\left(10\right)=10\text{ if }x\ge 0$

If we input a negative value, the output is the reverse of the input.

$f\left(x\correct)=-x\text{ if }ten<0$

Because this requires ii dissimilar processes or pieces, the absolute value part is an example of a piecewise function. A piecewise function is a office in which more than one formula is used to define the output over different pieces of the domain.

We use piecewise functions to depict situations in which a rule or relationship changes equally the input value crosses sure "boundaries." For example, we often encounter situations in business for which the cost per piece of a certain detail is discounted once the number ordered exceeds a certain value. Taxation brackets are another real-world example of piecewise functions. For instance, consider a simple tax organisation in which incomes upwards to $ten,000 are taxed at 10%, and any boosted income is taxed at xx%. The tax on a total income, Southward, would exist 0.1S if

${S}\le$

$10,000 and 1000 + 0.two (S - $ten,000), if Southward> $ten,000.

A General Notation: Piecewise Part

A piecewise function is a function in which more than one formula is used to ascertain the output. Each formula has its own domain, and the domain of the function is the matrimony of all these smaller domains. We notate this idea like this:

$f\left(ten\right)=\begin{cases}\text{formula 1 if ten is in domain 1}\\ \text{formula two if 10 is in domain 2}\\ \text{formula 3 if x is in domain iii}\end{cases}$

In piecewise notation, the absolute value function is

$|x|=\begin{cases}x\text{ if }ten\ge 0\\ -10\text{ if }x<0\stop{cases}$

How To: Given a piecewise office, write the formula and identify the domain for each interval.

1. Identify the intervals for which different rules apply.
2. Decide formulas that describe how to calculate an output from an input in each interval.
3. Use braces and if-statements to write the function.

Instance 11: Writing a Piecewise Function

A museum charges $5 per person for a guided bout with a group of 1 to 9 people or a fixed $fifty fee for a group of x or more people. Write a office relating the number of people,

$n$

, to the toll,

$C$

.

Solution

2 dissimilar formulas will be needed. For n-values under 10, C=5n. For values of n that are ten or greater, C=50.

C(n)=

$\brainstorm{cases}{5n}\text{ if }{0}<{n}<{x}\\ 50\text{ if }{n}\ge ten\end{cases}$

Analysis of the Solution

The function is represented in Effigy 21. The graph is a diagonal line from

$north=0$

to

$n=10$

and a constant afterwards that. In this case, the two formulas hold at the meeting betoken where

$n=10$

, but not all piecewise functions have this property.

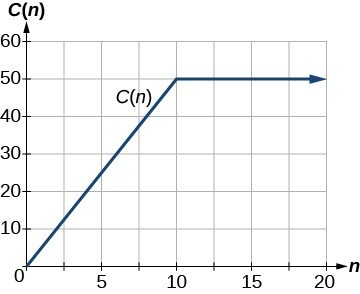Effigy 21

Instance 12: Working with a Piecewise Office

A cell telephone company uses the function below to make up one's mind the cost,

$C$

, in dollars for

$g$

gigabytes of data transfer.

$C\left(g\right)=\brainstorm{cases}{25} \text{ if }{ 0 }<{ grand }<{ ii }\\ { 25+10 }\left(g - 2\right) \text{ if }{ g}\ge{ 2 }\end{cases}$

Observe the cost of using i.5 gigabytes of data and the cost of using 4 gigabytes of data.

Solution

To find the price of using 1.v gigabytes of information, C(1.5), we first look to run across which part of the domain our input falls in. Because i.5 is less than 2, we utilize the first formula.

C(ane.five) = $25

To find the toll of using 4 gigabytes of data, C(iv), nosotros run across that our input of 4 is greater than 2, so we use the 2d formula.

C(4)=25 + 10( 4-2) =$45

How To: Given a piecewise function, sketch a graph.

1. Indicate on the x-axis the boundaries divers past the intervals on each piece of the domain.
2. For each slice of the domain, graph on that interval using the respective equation pertaining to that piece. Do not graph two functions over i interval because information technology would violate the criteria of a function.

Instance xiii: Graphing a Piecewise Role

Sketch a graph of the function.

$f\left(x\right)=\begin{cases}{ ten }^{two} \text{ if }{ x }\le{ i }\\ { iii } \text{ if } { 1 }&lt{ x }\le ii\\ { x } \text{ if }{ ten }&gt{ ii }\finish{cases}$

Solution

Each of the component functions is from our library of toolkit functions, so we know their shapes. Nosotros can imagine graphing each function and so limiting the graph to the indicated domain. At the endpoints of the domain, we describe open circles to indicate where the endpoint is not included because of a less-than or greater-than inequality; we draw a closed circumvolve where the endpoint is included because of a less-than-or-equal-to or greater-than-or-equal-to inequality.

Below are the 3 components of the piecewise function graphed on separate coordinate systems.

(a)

$f\left(ten\right)={x}^{2}\text{ if }ten\le 1$

; (b)

$f\left(x\correct)=three\text{ if 1< }x\le ii$

; (c)

$f\left(ten\right)=x\text{ if }x>ii$

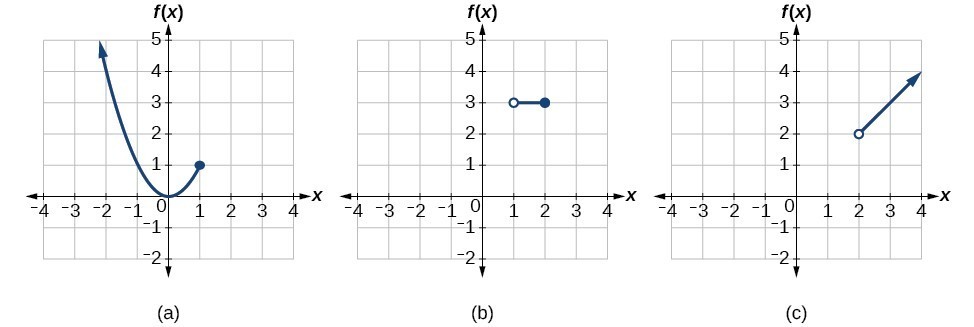Figure 23

Now that we have sketched each piece individually, we combine them in the same coordinate plane.

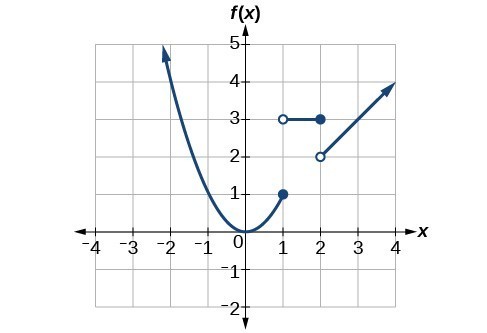Figure 24

Effort Information technology eight

Graph the following piecewise function.

$f\left(x\right)=\begin{cases}{ ten}^{3} \text{ if }{ x }&lt{-1 }\\ { -ii } \text{ if } { -1 }&lt{ x }&lt{ 4 }\\ \sqrt{ten} \text{ if }{ x }&gt{ 4 }\terminate{cases}$

Q&A

Can more than one formula from a piecewise function be practical to a value in the domain?

No. Each value corresponds to ane equation in a piecewise formula.

Licenses and Attributions

Source: https://www.coursehero.com/study-guides/ivytech-collegealgebra/graph-piecewise-defined-functions/

Posted by: perrytworiblest.blogspot.com

Share this post